Cauchy's integral formula states that

(1)

where the integral is a contour integral along the contour enclosing the point .

It can be derived by considering the contour integral

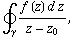(2)

defining a path as an infinitesimal counterclockwise circle around the point , and defining the path as an arbitrary loop with a cut line (on which the forward and reverse contributions cancel each other out) so as to go around . The total path is then

(3)

so

(4)

From the Cauchy integral theorem, the contour integral along any path not enclosing a pole is 0. Therefore, the first term in the above equation is 0 since does not enclose the pole, and we are left with

(5)

Now, let , so . Then

			(6)
			(7)

But we are free to allow the radius to shrink to 0, so

			(8)
			(9)
			(10)

giving (1).

If multiple loops are made around the point , then equation (10) becomes

(11)

where is the contour winding number.

A similar formula holds for the derivatives of ,

			(12)
			(13)
			(14)
			(15)
			(16)

Iterating again,

(17)

Continuing the process and adding the contour winding number ,

(18)

Arfken, G. "Cauchy's Integral Formula." §6.4 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 371-376, 1985.

Kaplan, W. "Cauchy's Integral Formula." §9.9 in Advanced Calculus, 4th ed. Reading, MA: Addison-Wesley, pp. 598-599, 1991.

Knopp, K. "Cauchy's Integral Formulas." Ch. 5 in Theory of Functions Parts I and II, Two Volumes Bound as One, Part I. New York: Dover, pp. 61-66, 1996.

Krantz, S. G. "The Cauchy Integral Theorem and Formula." §2.3 in Handbook of Complex Variables. Boston, MA: Birkhäuser, pp. 26-29, 1999.

Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 367-372, 1953.

Woods, F. S. "Cauchy's Theorem." §146 in Advanced Calculus: A Course Arranged with Special Reference to the Needs of Students of Applied Mathematics. Boston, MA: Ginn, pp. 352-353, 1926.

LAST MODIFIED: June 9, 2002

CITE THIS AS:

Weisstein, Eric W. "Cauchy Integral Formula." From MathWorld--A Wolfram Web Resource. http://mathworld.wolfram.com/CauchyIntegralFormula.html

© 1999 CRC Press LLC, © 1999-2007 Wolfram Research, Inc. | Terms of Use